integral of sinx cosx using integration by parts




What is the integral of cos(x) Ln(sin(x)) dx? - Yahoo! Answers.
Can someone here help with integration of e^3x(sinx)dx ? - Yahoo.
Answer: Integral of cos^2x=(1/2)(cosxsinx+x)+C Here is why: Here is one method : use integration by parts and let u=cosx and dv=cosxdx du=-sinx v=sinx.
Set your integral equal to I. You will have to use integration by parts twice at which. int[e^x(cosx)] = e^x(sinx) + (e^x)(cosx) - int[(e^x)(cosx)].
That is meant to be integral e^3x(sinx)dx but I doŌĆ”. Use integration by parts again. e^(3x).(3sin(x) - cos(x)) - 9I + k ŌåÉ constant of integration.
However, by the Fundamental Theorem of Calculus, the first integral on the right side. We use integration by parts when we see two distinct functions that don't seem to .. In fact, the only difference is that we have a sin(x) instead of a cos(x).
Integrate (dx) / ((sin x)^2 (cos x)) WITHOUT using integration by parts? 2 years ago; Report Abuse · Hemant by Hemant. Member since: August.
cos(x)ln(sin(x))dx we will use integration by parts:. =sin(x)ln(sin(x))- Ōł½ sin(x) ( cos(x)/sin(x). Evaluate the integral: Ōł½ (cosx) ln(sinx) dx?
Integration by Parts - HMC Calculus Tutorial.
Integration by parts of (e^x)(cos x) | Integration by. - Khan Academy.
How to find the integral of x sinx using integration by parts.
u=x v=sinx i do x*sinx then integrate v and differentiate u. -cos*1. v'=sinx u'=dx v =-cos(x) -xcos(x)- integral of -cos(x)dx -xcos(x)- -sin(x).
=2(integral)sin(x)cos(x)[e^cos(x)] Let z=cos(x) dz=-sin(x)dx thus our integral becomes =-2(integral)ze^z. Using integration by parts, u=z u'=1.
1) Use integration by parts twice, and then solve for the integral. Letting J. So, J = e^(4x) sin x + 4e^(4x) cos x - Ōł½ 16 e^(4x) cos x dx.
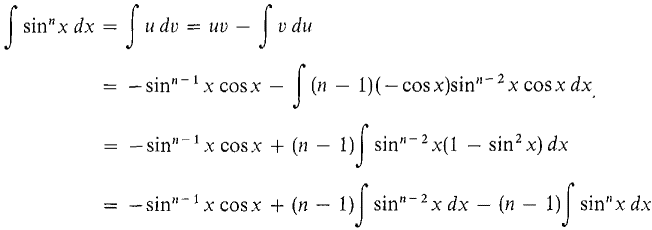
Antiderivative of xcosx using integration by parts · Integral of. At about 1:55, when he says integral of e^x*sin dx, should it be e^x*sinx dx instead? I think this is.
Using the trigonometric identity sin(2x). Ōł½(e^x)sin(x)cos(x) dx = (1/2) Ōł½(e^x)sin (2x) dx we can now integrate by parts. Let u = sin(2x) then.
Integral of cosine squared x - Wiki Answers.
What is the integral of x^2(sinx)dx ? - Yahoo! Answers.
u=x v=sinx i do x*sinx then integrate v and differentiate u. -cos*1. v'=sinx u'=dx v =-cos(x) -xcos(x)- integral of -cos(x)dx -xcos(x)- -sin(x).
=2(integral)sin(x)cos(x)[e^cos(x)] Let z=cos(x) dz=-sin(x)dx thus our integral becomes =-2(integral)ze^z. Using integration by parts, u=z u'=1.
Can anyone integrate e^x times sinx? - Yahoo! Answers.
Integration By Parts - UCSD.
Calculus Help: evaluate integral using integration by parts.
Answer: Integral of cos^2x=(1/2)(cosxsinx+x)+C Here is why: Here is one method : use integration by parts and let u=cosx and dv=cosxdx du=-sinx v=sinx.
Set your integral equal to I. You will have to use integration by parts twice at which. int[e^x(cosx)] = e^x(sinx) + (e^x)(cosx) - int[(e^x)(cosx)].